
Much of our work in Chapter 4 has been motivated by the velocity-distance problem: if we know the instantaneous velocity function, \(v(t)\text\)įigure 4.4.9. What is the meaning of the definite integral of a rate of change in contexts other than when the rate of change represents velocity? What is the statement of the Fundamental Theorem of Calculus, and how do antiderivatives of functions play a key role in applying the theorem? How can we find the exact value of a definite integral without taking the limit of a Riemann sum? Section 4.4 The Fundamental Theorem of Calculus Motivating Questions Population Growth and the Logistic Equation.Qualitative behavior of solutions to DEs.An Introduction to Differential Equations.Physics Applications: Work, Force, and Pressure.We will give the Fundamental Theorem of Calculus showing the relationship between derivatives and integrals.
#FUNDAMENTAL THEOREM OF CALCULUS FORMULA HOW TO#
We will discuss the definition and properties of each type of integral as well as how to compute them including the Substitution Rule.
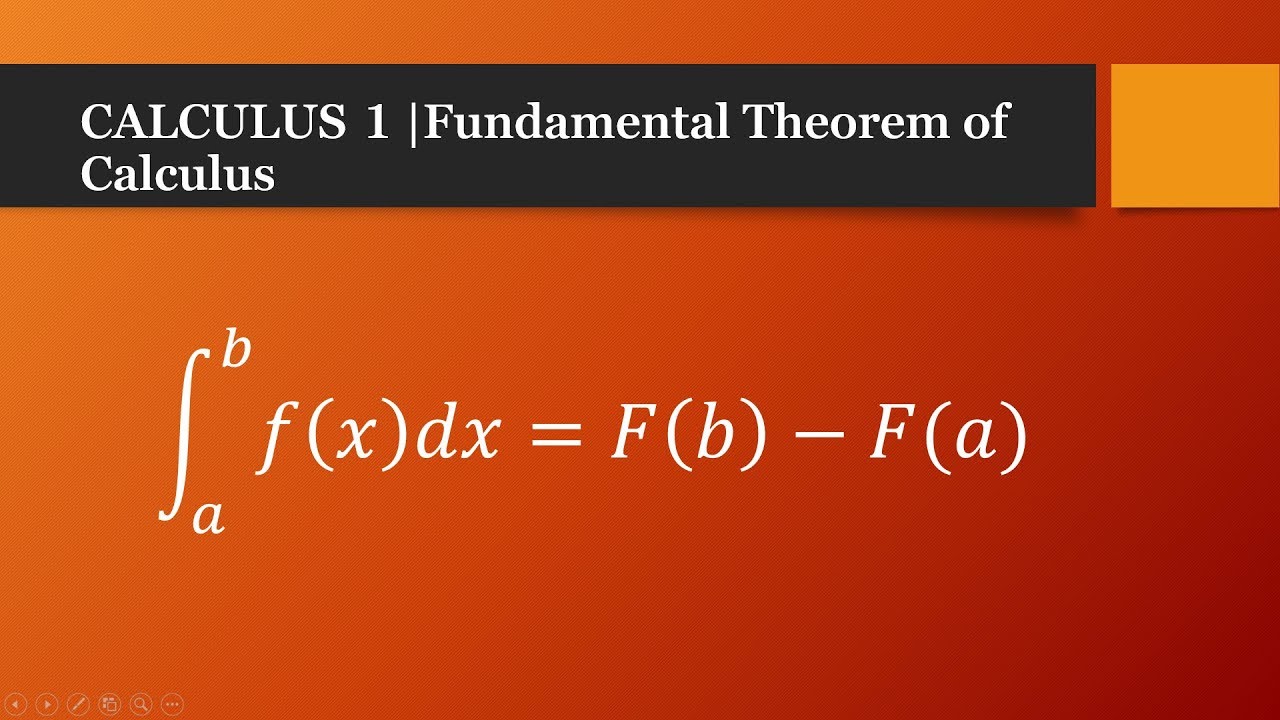
The derivative of a function at a point.Sample Questions Example 1įind F'(x), given F(x)=\int _ over the interval, with a=0. Here it is Let f(x) be a function which is dened and continuous for a x b. It converts any table of derivatives into a table of integrals and vice versa. That is, F'(x)=f(x).įurther, F(x) is the accumulation of the area under the curve f from a to x. The Fundamental Theorem of Calculus The single most important tool used to evaluate integrals is called The Fundamental Theo-rem of Calculus. Where F(x) is an anti-derivative of f(x) for all x in I. The Second Fundamental Theorem of Calculus defines a new function, F(x): The Definition of the Second Fundamental Theorem of CalculusĪssume that f(x) is a continuous function on the interval I, which includes the x-value a. The total area under a curve can be found using this formula.
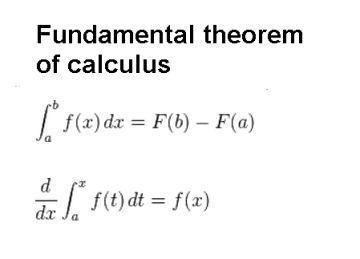
The Fundamental Theorem of Calculus, Part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand. So while this relationship might feel like no big deal, the Second Fundamental Theorem is a powerful tool for building anti-derivatives when there seems to be no simple way to do so. The Fundamental Theorem of Calculus, Part 1 shows the relationship between the derivative and the integral. The derivative of the integral, with respect to its upper bound, is the rate at which the. By this point, you probably know how to evaluate both derivatives and integrals, and you understand the relationship between the two. The integral of a function is the area under its curve (figure 1). When we do this, F(x) is the anti-derivative of f(x), and f(x) is the derivative of F(x). Specifically, for a function f that is continuous over an interval I containing the x-value a, the theorem allows us to create a new function, F(x), by integrating f from a to x.
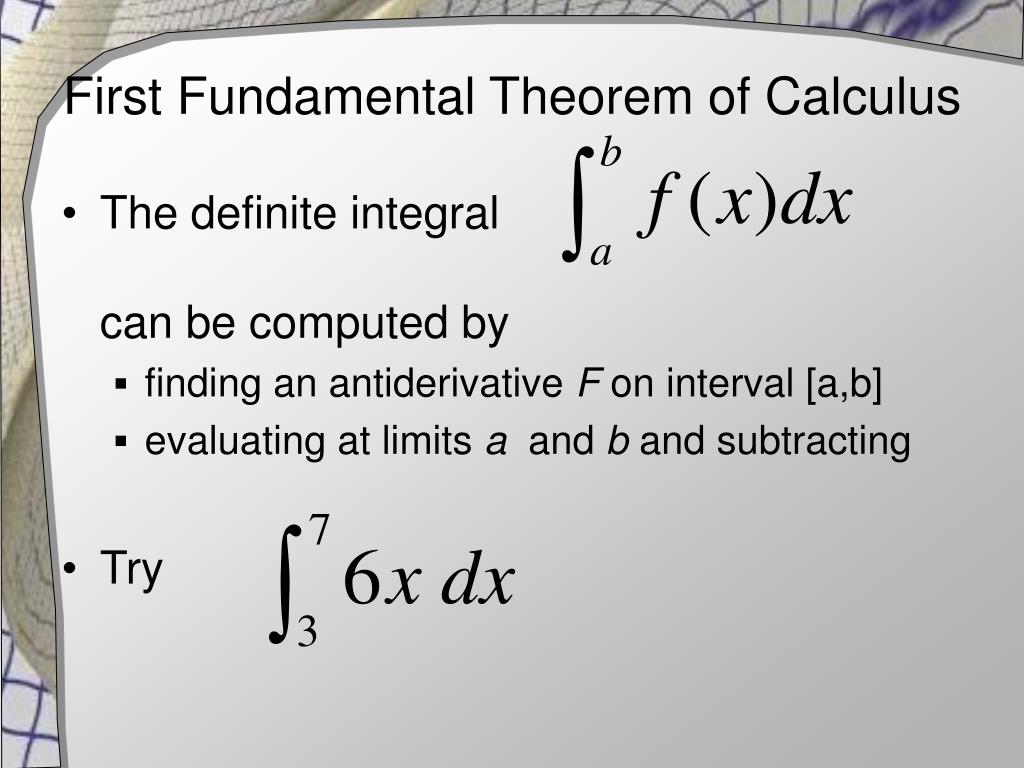
The Second Fundamental Theorem of Calculus establishes a relationship between a function and its anti-derivative.


 0 kommentar(er)
0 kommentar(er)
